SIGforum.com  Main Page
Main Page  Mason's Rifle Room
Mason's Rifle Room  Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
 Main Page
Main Page  Mason's Rifle Room
Mason's Rifle Room  Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.Go  | New  | Find  | Notify  | Tools  | Reply  |  |
| Freethinker |
I briefly mentioned this in another post, but I believe it may be of enough interest to warrant its own thread. One bit of marksmanship lore familiar to many shooters is that when shooting up or down at steep angles the point of impact of a shot will be higher than expected. And by “expected,” if we measure or estimate the direct distance from the shooter’s position to the target and use that range in our dope* data table to adjust our sight or point of aim, the bullet will hit higher than if we’d fired a horizontal shot at that same distance.† Below is my basic illustration from the other thread, but the distances were increased to make the differences clearer. Using the example triangle’s height and hypotenuse values, the horizontal distance to the target is 600 yards as indicated. The 22° down look angle is steeper than will be encountered in most long range shooting engagements, but not unreasonably so. 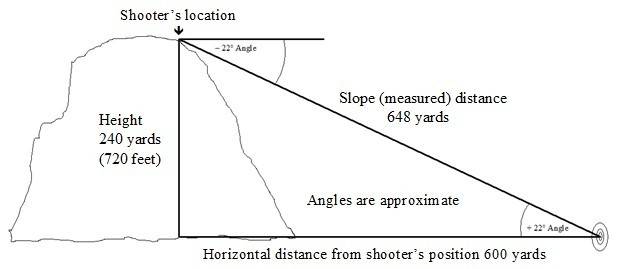 For this discussion I am using the Applied Ballistics solver under “standard” atmospheric conditions. The load is the Federal 168 grain 308 Winchester Gold Medal Match load at 2600 feet per second with a 210 yard zero and a sight height of 2 inches. (Changing the zero distance will change the absolute drop values at different ranges, but the trajectory differences among the values obtained for the various distances will be similar.) For starters, assume we have a laser rangefinder that measures the direct distance from the top of the cliff to the target as 648 yards. Using our ballistics dope data, it would tell us that we need to adjust our point of aim up 117.9 inches. (Yes, we wouldn’t be making adjustments in tenths of an inch, but bear with me; it’s to be exact in our examples. Also, the adjustments made to a scope sight setting would normally be in either milliradians or minutes of angle, but keeping this discussion in inches makes things clearer.) But let’s say our rangefinder will also use its angle sensor to measure a down angle of 22° and the direct distance measurement of 648 yards to give us the actual horizontal distance of 600 yards to the target as indicated by the base of our illustration triangle. If we use that distance and our dope table, it would tell us that the sight adjustment would be +93.4". So, which is it? Do we adjust up by 93.4 inches or 117.9"? The difference of over 2 feet (24.5") could result in a miss with some targets. The answer is that we should use our ballistics calculator to determine the actual point of aim adjustment necessary under the circumstances. Using the Applied Ballistics solver for the distance and angle involved, the actual adjustment necessary would be +105.7". That means if we use either the +93.4" or the +117.9" value, we’d be off from the true value by about a foot. Okay, why is there a difference between calculating the dope using either the 600 or 648 yard figure and what the solver tells us is correct for the situation? After all, the shooter is in just one location and the target is in another. The reason is that gravity affects the bullet for only the horizontal distance of 600 yards. Air drag, however, slows the bullet’s flight for the full 648 yards of atmosphere the bullet flies through. It’s therefore necessary to incorporate both effects in the calculations. Using the 600 dope doesn’t account for the additional air drag, and using 648 yards isn’t accurate for the gravity effect. In other words, using either the horizontal distance or the slope distance to the target will give us inaccurate results, and could result in a miss for that reason alone. That’s why a ballistics solver that allows incorporating an up/down shooting angle is important for best accuracy. As a final point about shooting under the conditions I described above, what if we don’t have a fancy ballistics solver at hand? There is a method called the “Improved Rifleman’s Method” (IRM) of correcting for extreme shooting angles. For that method we find the up/down shooting angle, find its trigonometric cosine, and multiply the slope distance dope data correction by the cosine number. For our example, the cosine of 22 degrees is about 0.93. Calculating: 117.9 × 0.93 = ~110 A drop correction of 110 inches is still not perfect, but now it’s about only 4 inches off from the ballistics solver value of about 106. To get all the values necessary for the calculations, they’re available on any scientific calculator and many smart phones. An even easier method, though, is to equip our rifle with a cosine angle indicator like the one shown at the lower left of this picture. The value it gives is the percentage to multiply our slant distance dope by to come up with the adjusted IRM value. With a 22° down angle, the indicator would show just over the 91 mark.  If you have gotten this far you may be asking what all that has to do with practical situations most shooters will find themselves in. If a military sniper were targeting a standing enemy soldier under the situation described and aimed at the center of the torso (and assuming the bullet went exactly where the dope table said it would), he could still be off by a foot and score a hit. And what about shallower angles and shorter ranges? I calculated that in the Mandalay Bay incident in Las Vegas if a law enforcement sniper had been on scene to target the killer it would have required shooting up a vertical distance of about 100 yards from a horizontal distance of about 400 yards. In that situation the up shooting angle would have been about 14 degrees. Using our example Gold Medal Match load, there would have been less than 2 inches difference between using either the horizontal or slope distance dope data and the actual aiming compensation adjustment required according to the ballistics solver. With shorter distances and shallower angles and with bullets having flatter trajectories than our 308 Winchester example, the sight adjustments necessary for the different distances become even less important, and would often be overwhelmed by variations in rifle precision and shooter skill. So, how much to we have to worry when shooting at an up or down angle? Not much. * And, no: “Dope” is not an abbreviation for “data on previous engagements.” If we have an accurate ballistics data set, it doesn’t matter if we have never shot at that range ever before in our lives. The term dope, meaning information about a variety of subjects, including sighting adjustments, was used long before anyone came up with that stupid contrived abbreviation. † Some commentators have stated that when viewing a target at an extreme up or down angle and estimating the range by eye, there may be a tendency to overestimate the distance, and that can also contribute to hitting higher than expected. This discussion isn’t about that possibility, though, and assumes accurate ranging by whatever means is used.This message has been edited. Last edited by: sigfreund, ► 6.0/94.0 To operate serious weapons in a serious manner. | ||
|
| Res ipsa loquitur |
Fascinating. As a deer hunter in the Rocky Mountains, this was a big debate as a kid. __________________________ | |||
|
Don't Panic |
Very cool! I don't shoot long distances but am curious about this aspect.
If I were back in freshman physics, where Mr. Newton ruled, I'd have said gravity acts on everything all the time, and the calculation I'd do to see how far it would be affecting the flight would be to calculate the time the projectile would be in free fall, which would be the time taken to go the sloped actual distance of 648 yards. Then take the duration of free fall and apply g (32 ft/sec/sec) over that time period and see the vertical deflection. I'm sure the ballistics calculator must be right, but what am I missing? | |||
|
| Freethinker |
I haven’t been ignoring your question. While being more involved in other activities than usual for me, I’ve been pondering it off and on and trying to decide what I know and how to explain it. I have never received any formal training on firearms ballistics, and therefore I run the autodidact’s risk of possibly misunderstanding or not being aware of obscure factors that affect the question. But with that as my cautious caveat, some discussion of what I do know I know. Saying that gravity affects the bullet’s flight for only the 600 yards may be misleading, and it’s certainly incomplete if it’s understood to mean that only gravity affects the bullet’s flight if shooting at a target on our same level (i.e., not up or down). As every shooter should know, it doesn’t matter what angle we shoot at, the bullet’s flight is slowed by atmospheric drag as it travels through the air. If we were shooting on the Moon or other body with gravity but no atmosphere, the bullet’s flight would be affected only by the gravity and its trajectory would be a simple parabola. That’s why (advanced) high school students may be asked to calculate a cannon ball’s trajectory, but the question will include something to the effect of “Ignore air resistance.” On Earth, however, we do have air drag and that complicates things. As a projectile travels through the air, it’s being pulled down by gravity, and the amount it’s pulled down in any distance is dependent upon the time it takes to travel that distance. That’s where your 32 feet per second per second comes in. What complicates ballistics calculations and keeps a projectile’s trajectory from being a simple parabola is that continuous incremental slowing due to air drag. The time it takes for a bullet to travel from 100 to 101 yards is more than it takes it to travel from 99 to 100 yards, and therefore the amount of its drop due to gravity is slightly more from 100 to 101 yards than it was when traveling from 99 to 100 yards. As just one example, using my 168 grain Gold Medal Match bullet’s trajectory, the bullet will drop about 8.7 inches when traveling from 100 to 200 yards, but when traveling from 800 to 900, it drops about 106.4 inches. The reason for its much greater drop in the same 100 yard distance is because it’s traveling so much slower and it’s therefore affected (pulled down) by gravity for so much longer.* At 200 yards its velocity is about 2203 feet per second, whereas at 900 it’s about 1072 fps. (All those figures are from my Applied Ballistics solver.) (And the higher the velocity, the greater effect of drag. From 100 to 200 yards the bullet loses about 196 fps, and from 800 to 900 only about 114 fps. The effect of air drag also varies with the ballistic coefficient of the projectile; the velocity of a bullet with a high BC decreases more slowly than one with a lower BC.) When considering my original example of shooting down at an angle of 22 degrees, it’s easy to see that the effect of drag would be greater when traveling through 648 yards of air than through 600 yards. Because the bullet is traveling slower at the end of 648 than at the end of 600, gravity has more time to affect its flight. If our calculation only looked at the end velocity after traveling through 600 yards of air, then it would be using a velocity figure that’s higher than it would be, and therefore give us a sight adjustment figure that’s too low to be accurate. I don’t know if all that addressed your question. It’s difficult to know for sure from a short Internet exchange. What we do know, however, and regardless of the validity of everything about my explanation and discussion, is that when shooting long distances up or down at steep angles it’s necessary to find a solution that falls between the horizontal distance and the slope distance to the target. * Added: And the bullet has also been pulled down by gravity for a time and therefore is dropping faster at that range than the 32 feet per second that it does at the beginning of its flight. I also edited this post a bit to emphasize that the shooting ranges have to be long and the angles have to be steep for the issue to be important.This message has been edited. Last edited by: sigfreund, ► 6.0/94.0 To operate serious weapons in a serious manner. | |||
|
Member |
We have to make similar calculations when firing artillery. Here's the intro to Chapter 8, FM 6-40). "In a situation where the target is not at the same altitude as the firing battery, the elevation determined from the tabular firing table may not achieve effects on the target. Site is a correction factor for a trajectory which is computed in such a situation. The VCO Vertical Control Operator) computes site using either a Graphical Site Table (GST) or manual computations. In order to understand site, a brief description of certain elements of the trajectory is necessary." "INITIAL ELEMENTS OF THE TRAJECTORY 8-1. Vertical Interval. The vertical interval (VI) is the difference in altitude between the unit (or observer) and the target or point of burst. (See figure 8-1 on page 8-2.) The VCO determines the vertical interval by subtracting the altitude of the unit or observer from the altitude of the target or point of burst. The vertical interval is determined to the nearest meter and is a signed value. 8-2. Angle of Site. The angle of site ( ∡SI) is a geometric angle which compensates for the vertical interval at a given range between the firing unit and the target. (See figure 8-1 on page 8-2.) The VCO determines the angle of site by dividing the vertical interval by the range (or distance) in thousands of meters and applying a correction factor to account for the conversion from meters to mils. The angle of site has a positive value when the target is above the base of the trajectory and a negative value when the target is below the base of the trajectory. The angle of site is determined to the nearest 0.1 mil and is a signed value. Angle of site carries the same sign as the VI. 8-3. Complementary Angle of Site. When angle of site is added to the elevation from the tabular firing tables it will impart a change on the trajectory. It is too simple to assume that the projectile fired at a higher elevation will simply go further or that one fired at a lower elevation will simply not go as far. For example, a projectile fired at a higher elevation will also experience a steeper angle of ascent, a higher maximum ordinate, and a steeper angle of descent as well as many other small changes. This is referred to as “trajectory non-rigidity.” The complementary angle of site (CAS) is an angle that is algebraically added to the angle of site to compensate for the non-rigidity of the trajectory." "Cedat Fortuna Peritis" | |||
|
| Member |
Yes, I realize it is a thing, of course. On the other hand, as a longtime hunter it’s normally not an issue. I mean, shots under 100 yards, angle difference usually much less. I just shot an anterless deer last Nov in IL, maybe 70 yards with the Knight muzzle-loader. I was on a higher ridge, deer bedded below, across the creek. The deer went 15 yards after shot. Yes, 600 yards is a whole different critter. I just have to plan to get closer. | |||
|
| Freethinker |
Yes, at close distances with firearms the difference between using the slope or horizontal distance to the target is very small even at steep shooting angles and can usually be ignored. Part of the reason I was prompted to post this was a discussion I had some time ago about law enforcement sniping in which the angle thing came up as a matter of possible concern. Although I was already convinced that under the circumstances being considered there was no need to worry about point of impact changes, I did the research to confirm my belief and was able to put it to rest. There are many myths and misunderstandings about shooting, and it’s just as important to know what does not matter as what does. ► 6.0/94.0 To operate serious weapons in a serious manner. | |||
|
| Member |
I’ve glanced through a few ballistics articles, it’s seems more than I could list can have minuscule effects. I couldn’t list them because I don’t know them all, air density & similar factors. Of course these may matter with precision long range shooting. Even simply how round pressures can change with temperature. There have been tests with rounds from the freezer compared to 70 degree ammo. Of course certain powders have more change than others. Getting back to the average hunter, no biggie. | |||
|
| Staring back from the abyss |
I remember taking hunter's safety as a kid. Back then, the rule of thumb that was taught was to aim a high uphill and a low downhill. Then I took physics in high school and realized that for pretty much any shot inside 300 yards (99.9% of hunting shots) it doesn't make any appreciable difference. ________________________________________________________ "Great danger lies in the notion that we can reason with evil." Doug Patton. | |||
|
| Sigless in Indiana  |
My rangefinder can be setup to display the HCD (horizontal component of distance) or total distance and then it will give the angle of inclination or declination which can then be fed into the ballistic program. For quick and dirty firing solutions, or for anything under 300 yards, or for police snipers, it likely is a moot point because the effect will be miniscule. For long range shooting, it becomes a factor. | |||
|
| Sigless in Indiana  |
I just ran some quick numbers through Strelok for a comparison. 800 yard shot with my 5.56 precision AR. 69 grain Sierra match king @2850 FPS Flat and level my correction for 800 yards from a 100 yard zero is: 8.2 mils of dialed or held elevation, which equates to 236 inches of correction at that distance. 30 degree incline (uphill shot): 6.9 Mils of dialed or held elevation, which equates to 200 inches of correction at that distance 300 yards for the same rifle and load flat and level: 1.1 mils which is 12 inches of correction 30 degree incline .8 mils which is 9 inches of correction Uphill or downhill, the effect is the same at both of those distances. If you were shooting extreme long range, there would probably be a measurable difference from uphill to downhill at extreme angles. | |||
|
| Freethinker |
Based on the number of views this thread has reportedly received, it’s evidently not a topic that interests many members, but for those who are, this is a further explanation of the “Improved Rifleman’s Method” (IRM) of estimating the proper adjustments necessary when shooting at long ranges and steep angles and when we don’t have a ballistics calculator to give us the best answer. The first step is to determine the actual slope distance to the target by using a rangefinder or other method such as a calibrated milling reticle. We use our dope chart to then determine the proper sight or hold adjustment necessary for that range. For this example, assume that the chart tells us to hold 5.0 milliradians or 15.0 minutes of angle* high for the distance ranged. In the example I used above I showed the calculations in inches, but scopes suitable for the purpose will have reticles calibrated in mils or MOA. Second, we determine the shooting angle to the target. Some rangefinders will give that as a readout, but there are various aids for reading angles available from precision clinometers† to simple protractor and string devices. For us, we find that the angle is a steep 40 degrees down. We could refer to a printed table of cosines or use small scientific calculator, but my smart iPhone has trigonometric functions on its calculator, and that would tell us the cosine (cos) of 40° is about 0.77. Now multiply the value of 5.0 mils or 15.0 MOA by the cosine value of 0.77. 5.0 × 0.77 = 3.85 (~38 0.1 ₥ clicks) or 15.0 × 0.77 = 11.55 (~46 1/4 MOA clicks) Note that for this process we’re using is the dope data for the slope distance. If your rangefinder measures the look angle and uses that along with the slope distance to find and display the horizontal distance, ignore the horizontal distance. It’s of no use in this process. Again, though, the entire process of measuring the angle to the target and determining its cosine is handled automatically by a weapon-mounted cosine value indicator, albeit not as precisely. If I were aiming down at an angle of 40 degrees, the indicator would read close to the “82” on the device. Measuring the angle exactly and doing the math gives more precise results, but the device eliminates the need for all that and may be close enough depending upon the target. For differences between shooting uphill or downhill, I have seen some when playing with a ballistics solver, but they are very small. The best way to see exactly how much is to do that experimenting with a calculator. And to reiterate, under the vast majority of conditions that shooters will encounter, all this will be of no practical concern. I post it, though, for those who might benefit from the discussion, if for no other reason than it’s on a gun forum and it’s about shooting. * Yes, I know they’re not equivalent, but they’re just examples. † https://www.google.com/search?...x-b-1-d&q=clinometer ► 6.0/94.0 To operate serious weapons in a serious manner. | |||
|
| Powered by Social Strata |
| Please Wait. Your request is being processed... |
|
SIGforum.com  Main Page
Main Page  Mason's Rifle Room
Mason's Rifle Room  Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
 Main Page
Main Page  Mason's Rifle Room
Mason's Rifle Room  Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.
Point of impact differences when shooting at up or down angles. Update 30Mar23 with further info re using angle cosines.© SIGforum 2025